Here are the answers with discussion for this Weekend’s Quiz. The information provided should help you work out why you missed a question or three! If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of Modern…
The Weekend Quiz – August 18-19, 2018 – answers and discussion
Here are the answers with discussion for this Weekend’s Quiz. The information provided should help you work out why you missed a question or three! If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of Modern Monetary Theory (MMT) and its application to macroeconomic thinking. Comments as usual welcome, especially if I have made an error.
Question 1:
Only one of the following propositions is possible (with all balances expressed as a per cent of GDP):
- A nation can run an external deficit and equal government surplus while the private domestic sector is saving overall.
- A nation can run an external deficit and equal government surplus while the private domestic sector is dissaving overall.
- A nation can run an external deficit and a larger government surplus while the private domestic sector is saving overall.
- None of the above are possible as they all defy the sectoral balances accounting identity.
The best answer is the second option – “A nation can run an external deficit and equal government surplus while the private domestic sector is dis-saving overall”.
This is a question about the sectoral balances – the government fiscal balance, the external balance and the private domestic balance – that have to always add to zero because they are derived as an accounting identity from the national accounts.
To refresh your memory the balances are derived as follows. The basic income-expenditure model in macroeconomics can be viewed in (at least) two ways: (a) from the perspective of the sources of spending; and (b) from the perspective of the uses of the income produced. Bringing these two perspectives (of the same thing) together generates the sectoral balances.
From the sources perspective we write:
(1) GDP = C + I + G + (X – M)
which says that total national income (GDP) is the sum of total final consumption spending (C), total private investment (I), total government spending (G) and net exports (X – M).
Expression (1) tells us that total income in the economy per period will be exactly equal to total spending from all sources of expenditure.
We also have to acknowledge that financial balances of the sectors are impacted by net government taxes (T) which includes all tax revenue minus total transfer and interest payments (the latter are not counted independently in the expenditure Expression (1)).
Further, as noted above the trade account is only one aspect of the financial flows between the domestic economy and the external sector. we have to include net external income flows (FNI).
Adding in the net external income flows (FNI) to Expression (2) for GDP we get the familiar gross national product or gross national income measure (GNP):
(2) GNP = C + I + G + (X – M) + FNI
To render this approach into the sectoral balances form, we subtract total net taxes (T) from both sides of Expression (3) to get:
(3) GNP – T = C + I + G + (X – M) + FNI – T
Now we can collect the terms by arranging them according to the three sectoral balances:
(4) (GNP – C – T) – I = (G – T) + (X – M + FNI)
The the terms in Expression (4) are relatively easy to understand now.
The term (GNP – C – T) represents total income less the amount consumed less the amount paid to government in taxes (taking into account transfers coming the other way). In other words, it represents private domestic saving.
The left-hand side of Equation (4), (GNP – C – T) – I, thus is the overall saving of the private domestic sector, which is distinct from total household saving denoted by the term (GNP – C – T).
In other words, the left-hand side of Equation (4) is the private domestic financial balance and if it is positive then the sector is spending less than its total income and if it is negative the sector is spending more than it total income.
The term (G – T) is the government financial balance and is in deficit if government spending (G) is greater than government tax revenue minus transfers (T), and in surplus if the balance is negative.
Finally, the other right-hand side term (X – M + FNI) is the external financial balance, commonly known as the current account balance (CAD). It is in surplus if positive and deficit if negative.
In English we could say that:
The private financial balance equals the sum of the government financial balance plus the current account balance.
We can re-write Expression (6) in this way to get the sectoral balances equation:
(5) (S – I) = (G – T) + CAD
which is interpreted as meaning that government sector deficits (G – T > 0) and current account surpluses (CAD > 0) generate national income and net financial assets for the private domestic sector.
Conversely, government surpluses (G – T < 0) and current account deficits (CAD < 0) reduce national income and undermine the capacity of the private domestic sector to add financial assets.
Expression (5) can also be written as:
(6) [(S – I) – CAD] = (G – T)
where the term on the left-hand side [(S – I) – CAD] is the non-government sector financial balance and is of equal and opposite sign to the government financial balance.
This is the familiar MMT statement that a government sector deficit (surplus) is equal dollar-for-dollar to the non-government sector surplus (deficit).
The sectoral balances equation says that total private savings (S) minus private investment (I) has to equal the public deficit (spending, G minus taxes, T) plus net exports (exports (X) minus imports (M)) plus net income transfers.
All these relationships (equations) hold as a matter of accounting and not matters of opinion.
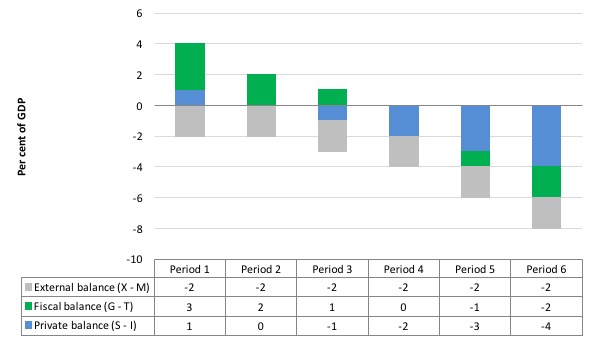
The following graph (with table) represents the three options in percent of GDP terms. To aid interpretation remember that (S-I) < 0 means that the private domestic sector is spending more than they are earning; that (G-T) < 0 means that the government is running a surplus because T > G; and (X-M) < 0 means the external position is in deficit because imports are greater than exports.
The first two possibilities we might call A and B:
A: A nation can run an external deficit and equal government surplus while the private domestic sector is saving overall.
B: A nation can run an external deficit and equal government surplus while the private domestic sector is dissaving overall.
So Option A says the private domestic sector is saving overall, whereas Option B say the private domestic sector is dissaving (and increasing indebtedness).
The private domestic sector only saves overall in Period 1 (S – I) = 1, when the external sector deficit of 2 per cent of GDP is accompanied by a larger fiscal deficit – (G – T) = 3.
The private domestic dissaves in Periods 3 to 6.
In each of those periods, the fiscal deficit is less than the the external deficit or in balance or surplus.
Given that the only proposition that can be true is:
B: A nation can run an external deficit and equal government surplus while the private domestic sector is dissaving overall.
The final option available is:
D: None of the above are possible as they all defy the sectoral balances accounting identity.
It cannot be true because as the Table data shows the rule that the sectoral balances add to zero because they are an accounting identity is satisfied in both cases.
So if the G is spending less than it is taxation receipts T and the external sector is adding less income (X) than it is absorbing spending (M), then the private domestic sector must be spending more than it is earning.
The following blogs may be of further interest to you:
- Barnaby, better to walk before we run
- Stock-flow consistent macro models
- Norway and sectoral balances
- The OECD is at it again!
Question 2:
The more funds that commercial banks have on account with the central bank the more they can lend to customers.
The answer is False.
This is a question about whether you understand the flawed concept of the money multiplier and bank reserves.
Mainstream macroeconomics textbooks present a flawed depiction of the credit-creation capacity of commercial banks. The concept of the money multiplier is at the centre of this analysis and posits that the multiplier m transmits changes in the so-called monetary base (MB) (the sum of bank reserves and currency at issue) into changes in the money supply (M). The chapters on money usually present some arcane algebra which is deliberately designed to impart a sense of gravitas or authority to the students who then mindlessly ape what is in the textbook.
They rehearse several times in their undergraduate courses (introductory and intermediate macroeconomics; money and banking; monetary economics etc) the mantra that the money multiplier is usually expressed as the inverse of the required reserve ratio plus some other novelties relating to preferences for cash versus deposits by the public.
Accordingly, the students learn that if the central bank told private banks that they had to keep 10 per cent of total deposits as reserves then the required reserve ratio (RRR) would be 0.10 and m would equal 1/0.10 = 10. More complicated formulae are derived when you consider that people also will want to hold some of their deposits as cash. But these complications do not add anything to the story.
The formula for the determination of the money supply is: M = m x MB. So if a $1 is newly deposited in a bank, the money supply will rise (be multiplied) by $10 (if the RRR = 0.10). The way this multiplier is alleged to work is explained as follows (assuming the bank is required to hold 10 per cent of all deposits as reserves):
- A person deposits say $100 in a bank.
- To make money, the bank then loans the remaining $90 to a customer.
- They spend the money and the recipient of the funds deposits it with their bank.
- That bank then lends 0.9 times $90 = $81 (keeping 0.10 in reserve as required).
- And so on until the loans become so small that they dissolve to zero
None of this is accurate in terms of depicting how the banks make loans. It is an important device for the mainstream because it implies that banks take deposits to get funds which they can then on-lend. But prudential regulations require they keep a little in reserve. So we get this credit creation process ballooning out due to the fractional reserve requirements.
The money multiplier myth also leads students to think that as the central bank can control the monetary base then it can control the money supply. Further, given that inflation is allegedly the result of the money supply growing too fast then the blame is sheeted home to the “government”. This leads to claims that if the government runs a fiscal deficit then it has to issue bonds to avoid causing hyperinflation. Nothing could be further from the truth.
That is nothing like the way the banking system operates in the real world. The idea that the monetary base (the sum of bank reserves and currency) leads to a change in the money supply via some multiple is not a valid representation of the way the monetary system operates.
First, the central bank does not have the capacity to control the money supply in a modern monetary system. In the world we live in, bank loans create deposits and are made without reference to the reserve positions of the banks. The bank then ensures its reserve positions are legally compliant as a separate process knowing that it can always get the reserves from the central bank. The only way that the central bank can influence credit creation in this setting is via the price of the reserves it provides on demand to the commercial banks.
Second, this suggests that the decisions by banks to lend may be influenced by the price of reserves rather than whether they have sufficient reserves. They can always get the reserves that are required at any point in time at a price, which may be prohibitive.
Third, the money multiplier story has the central bank manipulating the money supply via open market operations. So they would argue that the central bank might buy bonds to the public to increase the money base and then allow the fractional reserve system to expand the money supply. But a moment’s thought will lead you to conclude this would be futile unless (as in Question 3 a support rate on excess reserves equal to the current policy rate was being paid).
Why? The open market purchase would increase bank reserves and the commercial banks, in lieu of any market return on the overnight funds, would try to place them in the interbank market. Of-course, any transactions at this level (they are horizontal) net to zero so all that happens is that the excess reserve position of the system is shuffled between banks. But in the process the interbank return would start to fall and if the process was left to resolve, the overnight rate would fall to zero and the central bank would lose control of its monetary policy position (unless it was targetting a zero interest rate).
In lieu of a support rate equal to the target rate, the central bank would have to sell bonds to drain the excess reserves. The same futility would occur if the central bank attempted to reduce the money supply by instigating an open market sale of bonds.
In all cases, the central bank cannot influence the money supply in this way.
Fourth, given that the central bank adds reserves on demand to maintain financial stability and this process is driven by changes in the money supply as banks make loans which create deposits. Banks do not initially need reserves to lend. Reserves are used to facilitate the integrity of the clearing house (payments system). The links below provide more in-depth analysis of that point.
So the operational reality is that the dynamics of the monetary base (MB) are driven by the changes in the money supply which is exactly the reverse of the causality presented by the monetary multiplier.
So in fact we might write MB = M/m, where m is a divisor.
You might like to read these blogs for further information:
- Teaching macroeconomics students the facts
- Lost in a macroeconomics textbook again
- Lending is capital- not reserve-constrained
- Oh no … Bernanke is loose and those greenbacks are everywhere
- Building bank reserves will not expand credit
- Building bank reserves is not inflationary
- 100-percent reserve banking and state banks
- Money multiplier and other myths
Question 3:
Modern Monetary Theory (MMT) considers that the public debt ratio is of no concern because economic growth will always bring it down after a recession.
The answer is False.
First, the public debt ratio is of no concern per se if the nation is sovereign in its own currency. But the question was probing whether you understand the dynamics of the ratio in relation to GDP growth.
The primary deficit may not fall when economic growth is positive if discretionary policy changes offset the declining net spending as tax revenue increases and welfare payments fall (the automatic stabilisation).
Under current institutional arrangements, governments around the world voluntarily issue debt into the private bond markets to match $-for-$ their net spending flows in each period. A sovereign government within a fiat currency system does not have to issue any debt and could run continuous fiscal deficits (that is, forever) with a zero public debt.
The reason they is covered in the following blogs – On voluntary constraints that undermine public purpose.
The framework for considering this question is provided by the accounting relationship linking the fiscal flows (spending, taxation and interest servicing) with relevant stocks (base money and government bonds).
This framework has been interpreted by the mainstream macroeconommists as constituting an a priori financial constraint on government spending (more on this soon) and by proponents of Modern Monetary Theory (MMT) as an ex post accounting relationship that has to be true in a stock-flow consistent macro model but which carries no particular import other than to measure the changes in stocks between periods. These changes are also not particularly significant within MMT given that a sovereign government is never revenue constrained because it is the monopoly issuer of the currency.
To understand the difference in viewpoint we might usefully start with the mainstream view. The way the mainstream macroeconomics textbooks build this narrative is to draw an analogy between the household and the sovereign government and to assert that the microeconomic constraints that are imposed on individual or household choices apply equally without qualification to the government. The framework for analysing these choices has been called the government ‘budget’ constraint (GBC) in the literature.
The GBC is in fact an accounting statement relating government spending and taxation to stocks of debt and high powered money. However, the accounting character is downplayed and instead it is presented by mainstream economists as an a priori financial constraint that has to be obeyed. So immediately they shift, without explanation, from an ex post sum that has to be true because it is an accounting identity, to an alleged behavioural constraint on government action.
The GBC is always true ex post but never represents an a priori financial constraint for a sovereign government running a flexible-exchange rate non-convertible currency. That is, the parity between its currency and other currencies floats and the the government does not guarantee to convert the unit of account (the currency) into anything else of value (like gold or silver).
This literature emerged in the 1960s during a period when the neo-classical microeconomists were trying to gain control of the macroeconomic policy agenda by undermining the theoretical validity of the, then, dominant Keynesian macroeconomics. There was nothing particularly progressive about the macroeconomics of the day which is known as Keynesian although as I explain in this blog – Those bad Keynesians are to blame – that is a bit of a misnomer.
Anyway, just as an individual or a household is conceived in orthodox microeconomic theory to maximise utility (real income) subject to their fiscal constraints, this emerging approach also constructed the government as being constrained by a fiscal or “financing” constraint. Accordingly, they developed an analytical framework whereby the fiscal deficits had stock implications – this is the so-called GBC.
So within this model, taxes are conceived as providing the funds to the government to allow it to spend. Further, this approach asserts that any excess in government spending over taxation receipts then has to be “financed” in two ways: (a) by borrowing from the public; and (b) by printing money.
You can see that the approach is a gold standard approach where the quantity of “money” in circulation is proportional (via a fixed exchange price) to the stock of gold that a nation holds at any point in time. So if the government wants to spend more it has to take money off the non-government sector either via taxation of bond-issuance.
However, in a fiat currency system, the mainstream analogy between the household and the government is flawed at the most elemental level. The household must work out the financing before it can spend. The household cannot spend first. The government can spend first and ultimately does not have to worry about financing such expenditure.
From a policy perspective, they believed (via the flawed Quantity Theory of Money) that “printing money” would be inflationary (even though governments do not spend by printing money anyway. So they recommended that deficits be covered by debt-issuance, which they then claimed would increase interest rates by increasing demand for scarce savings and crowd out private investment. All sorts of variations on this nonsense has appeared ranging from the moderate Keynesians (and some Post Keynesians) who claim the “financial crowding out” (via interest rate increases) is moderate to the extreme conservatives who say it is 100 per cent (that is, no output increase accompanies government spending).
So the GBC is the mainstream macroeconomics framework for analysing these “financing” choices and it says that the fiscal deficit in year t is equal to the change in government debt (ΔB) over year t plus the change in high powered money (ΔH) over year t. If we think of this in real terms (rather than monetary terms), the mathematical expression of this is written as:
which you can read in English as saying that fiscal deficit (BD) = Government spending (G) – Tax receipts (T) + Government interest payments (rBt-1), all in real terms.
However, this is merely an accounting statement. It has to be true if things have been added and subtracted properly in accounting for the dealings between the government and non-government sectors.
In mainstream economics, money creation is erroneously depicted as the government asking the central bank to buy treasury bonds which the central bank in return then prints money. The government then spends this money. This is called debt monetisation and we have shown in the Deficits 101 series how this conception is incorrect. Anyway, the mainstream claims that if the government is willing to increase the money growth rate it can finance a growing deficit but also inflation because there will be too much money chasing too few goods! But an economy constrained by deficient demand (defined as demand below the full employment level) responds to a nominal impulse by expanding real output not prices.
But because they believe that inflation is inevitable if “printing money” occurs, mainstream economists recommend that governments use debt issuance to “finance” their deficits. But then they scream that this will merely require higher future taxes. Why should taxes have to be increased?
Well the textbooks are full of elaborate models of debt pay-back, debt stabilisation etc which all “prove” (not!) that the legacy of past deficits is higher debt and to stabilise the debt, the government must eliminate the deficit which means it must then run a primary surplus equal to interest payments on the existing debt.
Nothing is included about the swings and roundabouts provided by the automatic stabilisers as the results of the deficits stimulate private activity and welfare spending drops and tax revenue rises automatically in line with the increased economic growth. Most orthodox models are based on the assumption of full employment anyway, which makes them nonsensical depictions of the real world.
More sophisticated mainstream analyses focus on the ratio of debt to GDP rather than the level of debt per se. They come up with the following equation – nothing that they now disregard the obvious opportunity presented to the government via ΔH. So in the following model all net public spending is covered by new debt-issuance (even though in a fiat currency system no such financing is required).
Accordingly, the change in the public debt ratio is:
The change in the debt ratio is the sum of two terms on the right-hand side: (a) the difference between the real interest rate (r) and the GDP growth rate (g) times the initial debt ratio; and (b) the ratio of the primary deficit (G-T) to GDP.
A growing economy can absorb more debt and keep the debt ratio constant. For example, if the primary deficit is zero, debt increases at a rate r but the debt ratio increases at r – g.
So a change in the change in the debt ratio is the sum of two terms on the right-hand side: (a) the difference between the real interest rate (r) and the GDP growth rate (g) times the initial debt ratio; and (b) the ratio of the primary deficit (G-T) to GDP.
As we noted a growing economy can absorb more debt and keep the debt ratio constant. For example, if the primary deficit is zero, debt increases at a rate r but the debt ratio increases at r – g.
Consider the following table which simulates two different scenarios. Case A shows a real interest rate of zero and a steadily increasing annual GDP growth rate across 10 years. The initial public debt ratio is 100 per cent (so well over the level Reinhart and Rogoff claim is the point of no return and insolvency is pending). The fiscal deficit is also simulated to be 5 per cent of GDP then reduces as the GDP growth induce the automatic stabilisers. It then reaches a steady 2 per cent per annum which might be sufficient to support the saving intentions of the non-government sector while still promoting steady economic growth.
You can see that the even with a continuous deficit, the public debt ratio declines steadily and would continue to do so as the growth continued. The central bank could of-course cut the nominal interest rate to speed the contraction in the debt ratio although I would not undertake that policy change for that reason.
In Case B we assume that the government stops issuing debt with everything else the same. The public debt ratio drops very quickly under this scenario.
However, should the real interest rate exceed the economic growth rate, then unless the primary fiscal balance offsets the rising interest payments as percent of GDP, then the public debt ratio will rise.
The only concern I would have in this situation does not relate to the rising ratio. Focusing on the cause should be the policy concern. If the real economy is faltering because interest rates are too high or more likely because the primary fiscal deficit is too low then the rising public debt ratio is just telling us that the central bank should drop interest rates or the treasury should increase the discretionary component of the fiscal balance.
In general though, the public debt ratio is a relatively uninteresting macroeconomic figure and should be disregarded. If the government is intent on promoting growth, then the primary deficit ratio and the public debt ratio will take care of themselves.
You may be interested in reading these blogs which have further information on this topic:

Thank you for another illuminating quiz, Bill.
In a fiat currency system, the central bank does not control the money supply – the money supply is determined by the retail banks’ decisions about how much credit-worthy demand for loans exists in the private sector. Is that correct?
In a fiat currency system, the central bank does not control the monetary base either (aka the amount of high-powered money aka the amount of reserves) because the central bank makes an ongoing commitment to ensure that the retail banks have the reserves they need for payments to clear. So both the money supply and the monetary base are ultimately determined by the same thing: the retail banks’ decisions about how much credit-worthy demand for loans exists in the private sector. Is that correct?
Thank you for another illuminating quiz, Bill.
In a fiat currency system, the central bank does not control the money supply – the money supply is determined by the retail banks’ decisions about how much credit-worthy demand for loans exists in the private sector. Is that correct?
In a fiat currency system, the central bank does not control the monetary base either (aka the amount of high-powered money aka the amount of reserves) because the central bank makes an ongoing commitment to ensure that the retail banks have the reserves they need for payments to clear. So both the money supply and the monetary base are ultimately determined by the same thing: the retail banks’ decisions about how much credit-worthy demand for loans exists in the private sector. Is that correct?
That’s my understanding Nicholas. That probably is not worth much, but I really hope you are correct- because it would be difficult to re-read 1000+ posts here to figure out where it is wrong. Definitely rooting for your interpretation.
This is what I understand from Bill’s answer to Question 1.
These three things inject demand into the domestic private sector:
Government spending into the domestic private sector
Rest of the world spending on the domestic private sector’s output
Private sector dissaving (i.e. the private sector spends down its savings)
These three things drain demand from the domestic private sector:
Government taxation
Private sector spending on the rest of the world’s output
Private sector saving
The non-government sector includes the domestic non-government sector and the external sector (the external sector refers to the rest of the world, which includes foreign households, foreign firms, foreign banks, and foreign governments).
The financial balance of the government sector and the financial balance of the non-government sector (domestic and foreign combined) must sum to zero.
Therefore if the government sector is net draining demand from the domestic private sector (i.e. running a fiscal surplus) and the external sector is net draining demand from the domestic private sector (i.e. the rest of the world is running a current account surplus with regard to the domestic private sector), then by definition the domestic private sector must be dissaving overall.
This is the situation that Australia was in during the late 1990s and early 2000s during the Howard Government.
Is my understanding correct?
Thank you, Jerry! I appreciate your validation!
This is my understanding of what the term “non-government” means in the context of macroeconomic sectoral balances.
“Non-government” refers to any entity that merely uses the currency in question. So it includes subnational governments (such as state, provincial, local governments) and it includes foreign governments.
“Government” refers only to the government that issues the currency in question.
So if we are talking about sectoral balances denominated in the Australian dollar, “government sector” means the Australian Government, and the “non-government sector” includes:
Australian households
Australian firms
Australian banks
Australian non-bank financial institutions
state governments in Australia
local governments in Australia
foreign households
foreign firms
foreign banks
foreign non-bank financial institutions
foreign governments
If the entity merely uses the Australian dollar, instead of issuing it, then it is part of the non-government sector for macroeconomic accounting purposes. Even if the entity is a government of some description (i.e. a subnational government or a foreign government).
Does anyone agree?
Nicholas, I have asked this question also at one time. The response, according to my memory, was that the government sector includes everything we recognize as government- as in state and local governments. Even though they do not issue the currency. I am not sure it was Bill Mitchell that provided that answer though.
For what it is worth, I agree that the non-currency issuing subdivisions of government are very much like the private sector as far as MMT analysis is concerned. However, personally, I interact much more frequently with local and state government, as in pay them taxes, deal with their police, etc. And they are definitely ‘government’ – as in they can put me in jail or pull me over if I’m driving, or even shoot me if I got particularly unlucky. So I don’t know where to draw the line on what is the government sector.
Bill has been talking about how to ‘frame’ things for discussion lately. ‘Non-government sector’ is a very clunky terminology to use to describe what in the US is about 60% of our economy. It is accurate though and I really don’t have a good alternative.
I have two questions pertaining to answer 1:
(1) Once the term ‘(GNP – C – T)’ is labeled ‘private domestic saving’ and in the following paragraph as ‘total household saving’. Which is correct?
(2) Where is private consumption (as opposed to capital formation) in the expression: ‘(S – I)’?
Nicholas, the distinction is as you’ve identified – currency issuers vs currency users. So state and local governments are not in the Government sector of the equation.
Non Economist, private consumption is ‘I’ in (S – I). You could expand it further to say (Savings – (Consumption + Capital Formation). ‘I’ represents all spending.
Dear Non Economist (at 2018/08/20 at 2:36 am)
Thanks for your comment and enquiry.
1. The term (GNP-C-T) is household saving because it shows the residual disposable income households receive that they do not spend on consumption. It also shows private domestic saving. The confusion might be that in macroeconomics the term ‘saving’ refers to the unspent disposable income. I use the term ‘overall savings’ of the private domestic sector to include the retained earnings that businesses do not invest.
2. C nets out. To simplify (using a closed economy), GDP (Y) = C + I + G (the sources of expenditure).
That has to be equal to the uses of Y which are equal to C + S + T (where the Y goes).
So C + I + G = C + S + T
And C cancels out to give the usual simplified expression that the injections of spending (I + G) must equal the leakages (S + T) for Y to be stable.
I hope that helps.
best wishes
bill
I`ve been meaning to ask the following for some time:
When doing the multiplier, I usually determine “overall saving” then arrive at final consumption.
e.g. S = I + Fiscal(B) + CAB …. therefore Yd – S = C
I don`t but does anyone see a problem with that?
Dr. Mitchell, if the non-government sector cannot increase net financial assets without the cooperation of the government, then how should we think about equity shares issued by corporations? I understand that they have both an asset (the share) and a liability (an entry on the company’s balance sheet as a liability), but in reality, of what significance is the liability on the balance sheet?
On a bank’s balance sheet, loan assets and deposit liabilities are regularly created and regularly extinguished. For a corporation, the share assets and balance sheet liabilities are created and rarely, if ever, extinguished. In addition, the obligations that the corporation has to honor for the liability created are practically non-existent, unlike a bank’s obligation to honor the deposit liability it created.
So would you agree that shares are a way of the non-government sector to create net financial assets? I think the answer is ‘false’ but the logic escapes me.
Thanks, Joel
Joel S, I am obviously not Dr. Mitchell, but would like to state my understanding of your question. And if it is wrong I hope to be corrected. Because I am far from sure this is correct.
Start with something simple- I own a house and rent it out. You purchase an equity share of that house from me, say 30%. I don’t see that there is any liability created for me, or any net asset created for you by that share purchase. All you have done is purchase 30% of the (potential) sale value of the house and 30% of any profits from the rents. And that is what I have given up in exchange for your purchase of equity in the house. Net zero.
So lets say the sale value of the house decreases by 10%. I do not have any liability to you because of that- you are a co-owner and we both lose. The opposite if it increases in market price, but still no liability on my part.
Yeah, I hope Bill answers your question.
Hi Joel
My attempt to answer your question is that when you buy shares, you gain one financial asset (an equity stake in a firm) and relinquish another financial asset of equal nominal value (a demand deposit at a retail bank). The firm that issues the shares gains one financial asset (a demand deposit at a retail bank) and relinquishes another financial asset of equal nominal value (an equity stake in the firm). So all of the financial transactions net to zero.
Jerry Brown and Nicolas,
Thank you for your responses, but I can’t reconcile your answers with my question.
If a company issues stock, it has created a financial asset, adding to the stock of financial assets in the economy. If I understand Dr. Mitchell, the non-government sector cannot create net financial assets, so there must be a corresponding liability somewhere in the economy.
And there is! The balance sheet of the issuing company has a liability of ‘shares issued’. So in principle, Dr. Mitchell’s thesis holds, that the non-government sector cannot add net financial assets to the economy.
But – that liability on the balance sheet of the company is (from my opinion) functionally useless. Companies have little need to honor the liability (unlike a bank that needs to honor their deposit liability, for example).
So – if the share asset is matched by a (functionally useless) balance sheet liability, then the company has effectively created net financial assets! The shares have value. Wouldn’t this conclusion fly in the face of the MMT thesis that the non-government sector cannot create net financial assets?
But Nicolas,
I understand stand your logic, but consider:
Before the company existed, the stock of net financial assets is some amount say $100.
After the company issues shares, the stock of net financial assets in the non-government sector is $100 + the price of the added shares.
Is this correct?
Jerry Brown,
Thanks for the response, but I am not sure if your scenario is valid. Hasn’t the equity stake in the business created a financial asset where none existed? Isn’t that by definition creating net financial assets?
There is a similar question in this weeks’ quiz;I will post there to try and increase the visibility of my question.
Thanks, Joel
Joel, I am not sure. Supposing you did buy 30% of that house from me- I guess the sale contract or your inclusion on the property deed does become a financial asset for you if you were able to resell your stake to a third party without selling the real asset behind it (the house), so I think you are right there. But let me ask why would you just pay me for this new financial asset if you did not think that it obligated me in some way to you? At the very least it would give you the right to 30% of the proceeds if the property were sold. That is an obligation or liability on me. Maybe you can argue that the liability does not equal the new asset created, but the question of why you would pay for it if that were the case still remains.